EE3901/EE5901 Sensor Technologies Week 7 NotesCapacitive sensors and signal conditioning circuits for reactive sensors
Changes in capacitance provide another mechanism for electrical sensing. This week we will discuss some common capacitive sensors, as well as the interface circuits that are used to measure changes in capacitance.
Capacitance
Firstly, let’s review the physics of capacitors. A capacitor consists of two electrodes separated by an insulating material. An accumulation of charge on the electrodes creates an electric field between the plates. The electric field causes the effects we know as capacitance.
Capacitance is measured in farads (F), which is equivalent to coulombs/volt. In other words the capacitance tells you how much electric charge will accumulate on each electrode per volt.
Suppose we have rectangular electrodes, as shown in Figure 1.
The basic geometry of plane-parallel capacitors. There are two conductive electrodes (yellow) separated by an insulating material (transparent grey). The electrodes have surface area
These are called “plane parallel” electrodes. These serve as a prototypical example of a capacitor, and serve to explain most of the important physics.
The capacitance of plane parallel electrodes is given by
where
where
Fringing effects
The geometric capacitance (
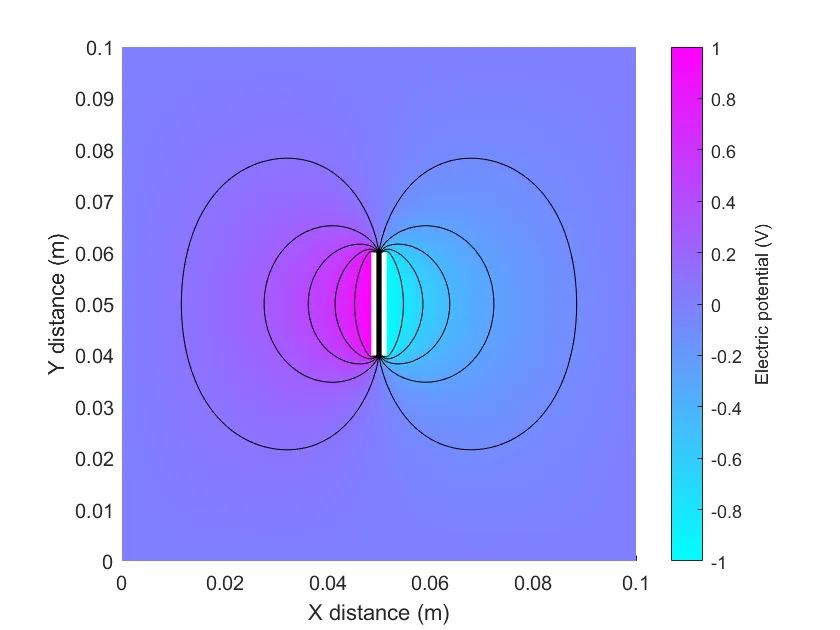
The calculated electric potential in the vicinity of plane parallel electrodes. This is a slice through the middle of a capacitor. The electrodes are the white rectangles, and you should visualise them as being extruded into the page. The left and right electrodes are connected to
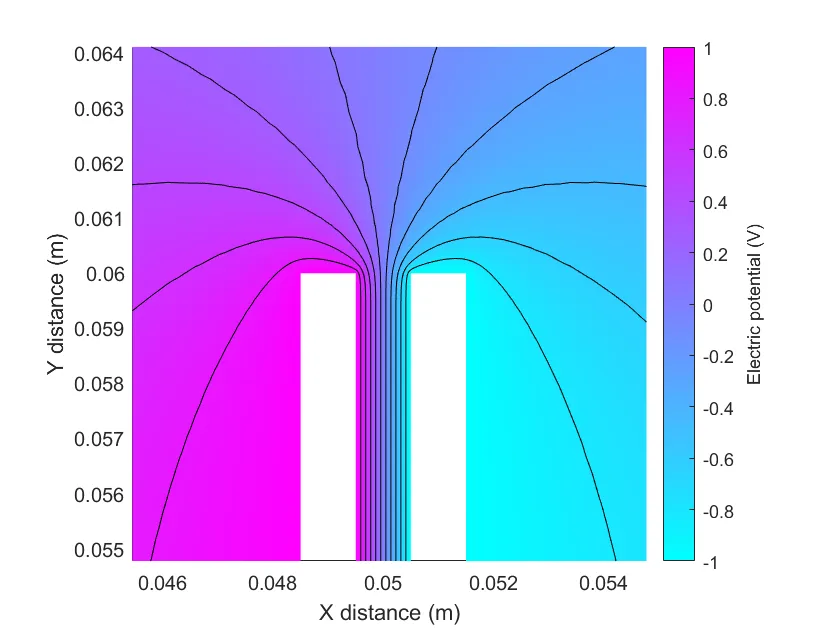
A zoomed in version of Figure 2, showing the region of fringe effects at the edge of the electrodes.
Zoom: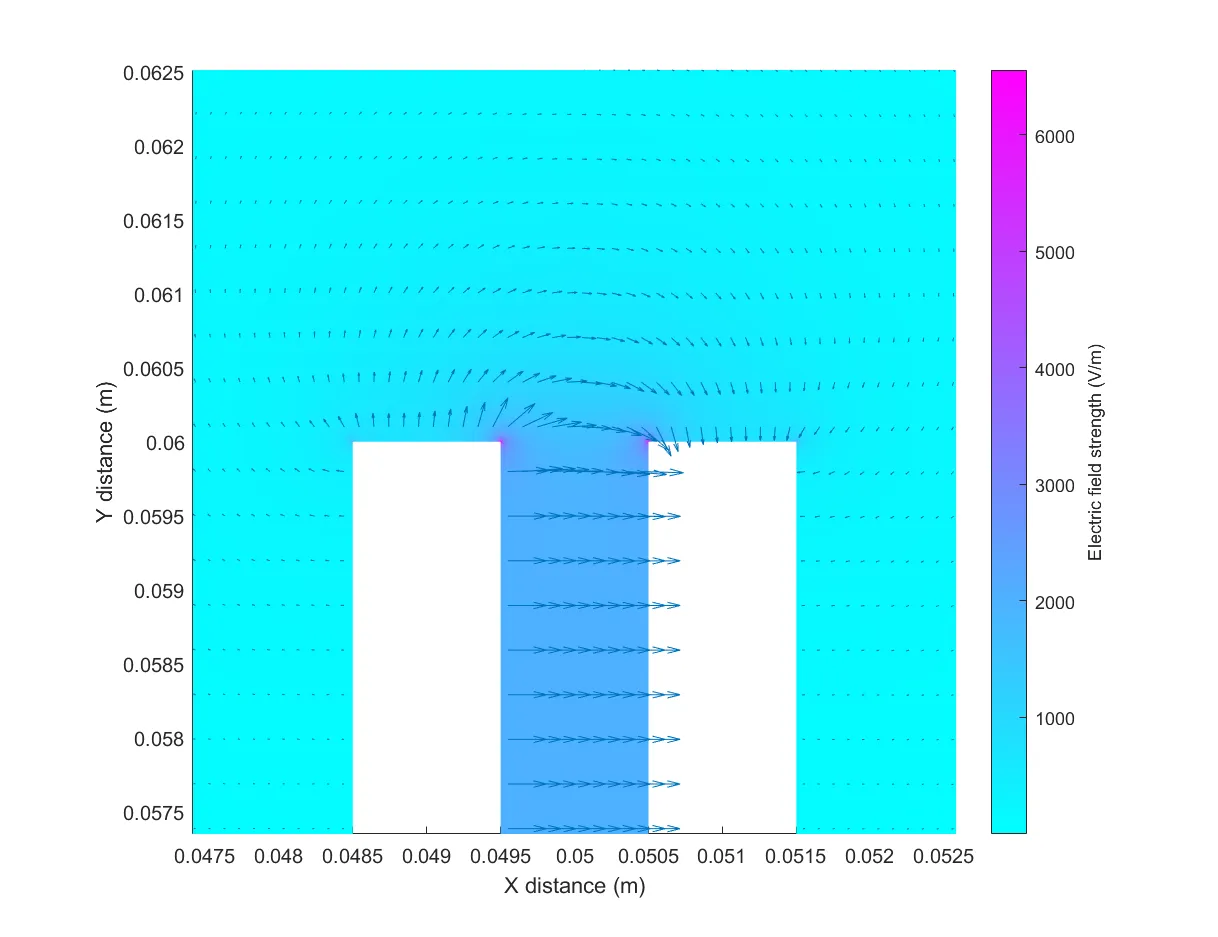
The electric field for the same capacitor as Figures 2-3. Notice the uniform electric field between the plates, the strong electric fields at the corners of the electrodes, and the fringing fields that weakly extend outside the capacitor’s main body.
Zoom:As shown in Figure 4, the electric field lines curve outwards near the edge of the capacitor. If this system were a sensor, and our goal was to measure changes in capacitance, these fringing effects may cause interference. For sensing purposes, we can minimise the edge effects by using guard plates (Figure 5).
A capacitor with split electrodes. The sensing electrode is the central plate shown in red. The adjacent electrodes are energised in the same manner, so that the neat parallel electric field lines are preserved in the vicinity of the sensing electrode.
Zoom:Using these guard electrodes, the capacitance between the middle electrodes is closer to the ideal geometric value,
Mechanisms of capacitance sensing
Using the equation
- Changes in area (e.g. by moving the electrodes sideways so that the area of overlap varies); or
- Changes in separation distance (e.g. by moving the electrodes closer together or further apart); or
- Changes in permittivity (e.g. by changing the properties of the material between the plates).
When we consider also the equivalent circuit of multiple capacitors, we can identify another mechanism:
- Introducing or removing nearby objects that are capacitively coupled to the sensor, thereby adding or removing a capacitors from the equivalent circuit of the sensor.
Some mechanisms are shown in Figure 6.
Examples of how capacitance can be used as a sensing mechanism. (a) Movement of electrodes causes variation in overlap area. (b) Capacitance is highly sensitive to the separation distance, so a common mechanism is to vary the distance between electrodes by moving or stretching the materials. (c) A dielectric material can be moved or undergo changes to its permittivity, for example, in a humidity sensor by absorbing water. (d) Capacitive touch screens operate by detecting the formation of new capacitive coupling.
Zoom:Capacitive humidity sensors
Capacitance is a useful mechanism to detect water because water has a high permittivity. (This is because water molecules are polar, and can easily orient themselves in response to an applied electric field.) The relative permittivity of air is
A typical mechanism is to use some absorbent material that takes up moisture from the air. A properly chosen absorbent polymer film can be used to create an almost linear relationship between capacitance and relative humidity. However, the permittivity varies with temperature, so the temperature must also be measured and corrections applied.
Capacitive level sensors
Capacitance can be used to measure the level of liquid, as shown in Figure 7.
The concept of water level sensing. (a) Capacitive electrodes are inserted in or near the liquid. (b) Electrically, this behaves like the parallel connection of many capacitors.
Zoom:The electrodes may have various geometries, for example, they may be cylindrical with an inner electrode and an outer electrode where the liquid travels up the middle like a pipe. Alternatively they may have flat electrodes arranged as parallel plates or coplanar plates. If the electrodes are inside the liquid, then they must be covered with an insulating material. Alternatively, the electrodes may be placed on the outside of the container walls.
Since the air and liquid have different permittivity values, the capacitance per unit length in the air and liquid region are different. As the water level rises, the proportion of the capacitor with a high permittivity increases, resulting in a close to linear increase in capacitance.
Again there is a temperature dependence that must be accounted for. One strategy to do this is to include two more electrode pairs to measure the capacitance of the liquid and air. This can be used to correct for changes in temperature.
Other applications
- Capacitance can be used to measure small displacements by moving the electrodes with respect to each other. For example some accelerometers measure the deflection of a proof mass suspended on a miniature spring.
- Studio condenser microphones measure the deflection of a diaphragm through change in capacitance.
- An electrically conducting object can be used as one electrode in a single ended capacitance probe.
Capacitive interface circuits
Interface circuits for capacitive sensors often use a sinusoidal voltage
or current. In this case, the capacitive sensor can be represented
by a complex impedance
For some intuition, consider a capacitance sensor with
However, there are also various circuit designs that are more specialised to capacitive sensor interfacing.
The auto-balancing bridge
The auto-balancing bridge (Figure 8) is a simple circuit that is common in LCR meters. To understand this circuit, notice that the op-amp creates a virtual ground at
The auto-balancing bridge circuit for capacitance measurement. This design is suitable for measurement frequencies below about 100 kHz. At higher frequencies, op-amps do not have enough gain-bandwidth and so different circuit designs are needed. (For more details, a good reference is the Keysight Technologies Impedance Measurement Handbook.)
Zoom:Op-amp circuits with linear responses
When impedance is linearly proportional to the measurand
Consider a capacitive sensor where the separation distance is varied. Specifically, consider that the distance is given by
where
where
Now consider the impedance of this capacitive sensor:
Notice that impedance is linearly proportional to the measurand. A suitable interface circuit is shown in Figure 9.
An op-amp based circuit where the output voltage is linearly proportional to the sensor’s impedance.
Zoom:The resistor
where
Notice that the output is linear with respect to
When impedance is inversely proportional to the measurand
Other types of capacitive sensors may have variations in permittivity or surface area, i.e. a response of the form:
In this case the capacitor’s impedance is
i.e. impedance is inversely proportional to the measurand. In this case, swap the placement of the variable and fixed capacitors:
An op-amp based circuit where the output voltage is inversely proportional to the sensor’s impedance. Equivalently, the output voltage is directly proportional to the sensor’s admittance.
Zoom:The resulting output voltage is
Again notice that the output voltage is linearly proportional to
AC bridges
Bridge circuits can also be used for capacitive sensors, as shown in Figure 11.
A generalisation of the Wheatstone bridge, used to measure the AC impedance of a sensor.
Zoom:The analysis is similar to the Wheatstone bridge except that impedances are used instead of resistances.
A variation of this concept is the Blumlein bridge, where a centre-tapped transformer is used on one side (Figure 12).
The Blumlein bridge circuit is a variant of the Wheatstone bridge where one side is a centre-tapped transformer. At least one of the impedances
The advantage of this design is that the sensing side has galvanic
isolation from the excitation source, so that it can be separately
grounded. Notice that
To analyse this circuit, notice that the action of the transformer
is to produce a voltage source with magnitude
Circuits based on charging & discharging capacitors
Transformers cannot be miniaturised, hence other interfacing circuits have been developed for integrated circuits. Instead of using sinusoidal excitation, capacitance can also be measured based on its charging and discharging behaviour.
Charge transfer
Charge can be transferred from an unknown capacitor to a known capacitor (Figure 13).
Simplified circuit diagram showing the principle of capacitance measurement by transferring charge between capacitors.
Zoom:This circuit operates in three steps:
- The unknown capacitor
- The energy stored in
The output voltage can be derived by conservation of charge. You will show in tutorial questions that
Variable frequency oscillators
An oscillator is a circuit that produces a periodic waveform. A common strategy to measure capacitance is to design an oscillator circuit whose output frequency depends upon the capacitance of the sensor. An example is the relaxation oscillator that uses a comparator:
The relaxation oscillator circuit, which is an capacitance measurement circuit suitable for frequencies up to tens of kHz.
Zoom:The sensor can be either
As drawn, this circuit is for a comparator with push-pull outputs,
for example the TLV3491.
The pullup resistor
Other types of oscillators (e.g. Wein bridge oscillators, Hartley oscillators, and Colpitts oscillators) operate at higher frequencies, and hence can be used with sensors whose capacitance is smaller. The principle is the same: changes in capacitance cause changes in oscillation frequency, and the frequency is then measured with a microcontroller or other digital circuit.
Conclusion
Capacitance is commonly used for sensing purposes because it enables relatively long range, contactless measurements. The most famous example of capacitive sensing is the touchscreen found in many portable electronics devices. Capacitance is also common for sensing the presence or absence of various materials, for humidity sensing, and more.
There are two main types of interface circuits for capacitive sensors. In many designs, an AC voltage source is used to treat the capacitance as a complex impedance, and the impedance measured using similar methods to that of resistive sensors. In other designs, changes in capacitance control the timing of an oscillator or the time constant of an RC circuit.
References
Ramon Pallas-Areny and John G. Webster, Sensors and Signal Conditioning, 2nd edition, Wiley, 2001.
Winncy Y. Du, Resistive, Capacitive, Inductive, and Magnetic Sensor Technologies, CRC Press, 2015.